Assume that at t=-∞ a system is in an eigenstate |φi> of the Hamiltonian H0. At t=t1 the system is perturbed and the Hamiltonian becomes H=H0+W(t).
When investigating the interaction picture we found that the probability
of finding the system in the eigenstate
to first order in the perturbation W.
We often write
This is the result of first
order time dependent perturbation theory.
 |
Assume that W(t) = W⋅sinωt, i.e. that we have a sinusoidal perturbation starting at t=0. Then |
|
Similarly, if W(t) = W⋅cosωt, then |
|
If ω = 0 we have a constant perturbation and |
For the harmonic perturbation W(t) = W⋅sinωt, we find that
has an appreciable amplitude only if the denominator of one of the two terms is approximately zero, i.e. if
The first order effect of a perturbation that varies sinusoidally with
time is to receive from or transfer to the system a quantum of energy 
If the system is initially in the ground state, then Ef>Ei, and only the second term needs to be considered. Then
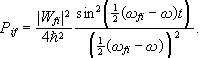
Let β = ωfi - ω, and plot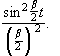 
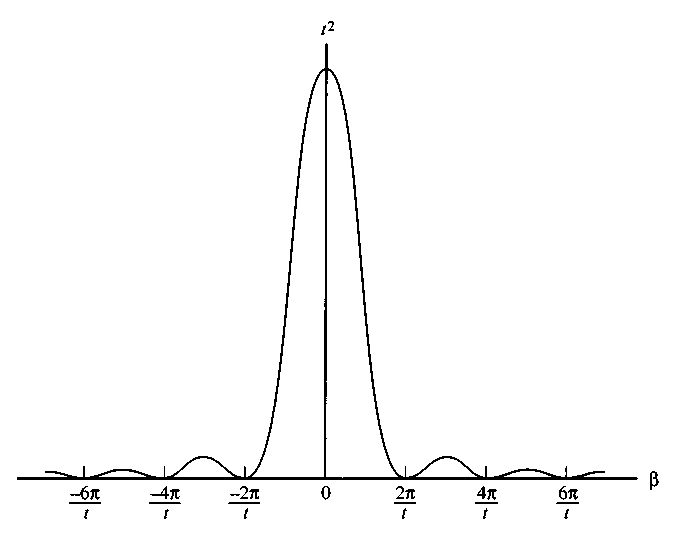
If β = 0, i.e. ωfi = ω , then Therefore, if ωfi = ω, then the probability of finding the system in the state |φf>, In the above figure the
height of For a first order approximation
to be valid, we need On the other hand, to justify
neglecting the first term in the above formula, we need 2ωfi>>Δω. 2ωfi is the difference in the positions of the peaks due to the first term and the second term in the above formula, Δω is the width of the peaks, Δω ≈4π/t.
We therefore need
Combining these two conditions
we obtain
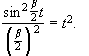
increases linearly with time.
increases
proportional to t2, and the width of the peak is proportional
to 1/t. The area under the curve is proportional to t.
Assume there is a group
of states n, nearly equal in energy E, and that Wni = <φn |W| φi > is nearly independent of n for these states. Take for example continuum states. We may label continuum states by |α>, where α is continuous. <α|α> = δ(α-α'). The probability
of making a transition to one of these states in a small range Δα is 
If | α> = | β,E> then dα = ρ(β,E)dE,
where
If W is a constant perturbation, then
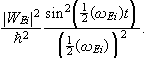
The function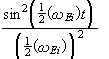 
peaks
at ωEi = 0 and has an appreciable amplitude only in a small interval ΔωEi or ΔE about ωEi = 0. We assume that ρ(β,E)
and |WEi|2 are nearly constant in that small
interval and therefore may be taken out of the integral. Then
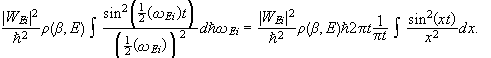
Therefore,
It is understood that the expression is integrated with respect to dE.
The transition probability per unit time is the given by Fermi’s golden rule,
Similarly, for a sinusoidal perturbation W(t)=Wsinωt or W(t)=Wcosωt we obtain
and for W(t)=Wexp(±iωt) we obtain
We have 
(Coulomb
or radiation gauge)
We have a plane electromagnetic wave propagating in the y-direction.
Let 
and 
with
E0 and B0 real, 
(
SI units ) Then
S is the Poynting vector.
The Hamiltonian of an atomic electron interacting with this plane wave is
In this expression we consider only one independent electron, and we neglect the spin orbit interaction. We have
[pz,Az]=0 since Az depends only on y.
To find induced transition probabilities, we have to evaluate the matrix elements of W(t) between unperturbed bound states. An order of magnitude estimate reveals
in the optical domain. We assume that the intensity of the wave is low enough so that WIII, the term containing A02, can be neglected compared to terms containing A0.
since
y is on the order of atomic dimensions.
Let WDE(t) be the zeroth order term in the expansion.
WDE is called the electric dipole Hamiltonian. The electric dipole approximation assumes W(t)=WDE(t).
Note: This form of WDE is equivalent to the form we would get starting with the energy of an electric dipole in an electric field.
This equivalence can be
shown via a gauge transformation 
The matrix elements of WDE are proportional to the matrix elements of z, because E is in the z direction.
The integrant is a product of three spherical harmonics and the integral can be given in terms of Clebsch Gordan coefficients.
These are coefficients 
The integral is zero unless
|
i)  |
|
ii)  |
|
iii)  |
since 
We therefore have that 
unless 
If we choose another direction for the polarization of E,
i.e. 
then
we find 
The dipole transition selection rulestherefore are
These selection rules result as a consequence of the properties of the spherical harmonics.
An electromagnetic field is most likely to induce a transition between an initial and a final state if these selection rules are satisfied. If these selection rules are not satisfied a transition is less likely and is said to be forbidden.
When deriving the dipole
transition selection rules Δl=±1,
Δm=0,
±1, we assumed that the Hamiltonian 
was
perturbed by WDE(t).
We neglected the spin orbit interaction.
If H0 contains a spin orbit coupling term f(r)L·S,
then the eigenstates of H0 are
{|l,s;j,mj>} and not {|l,s;m,ms>}. The dipole selection rules then become Δj=0, ±1, (except ji=jf=0), Δl=±1, Δmj = 0, ±1.
(p or r are vector operators. The selection rules follow from the Wigner-Eckart theorem.)
if we take the first order
term in the expansion of exp(±iky). We may write 
If in the expression for
WII(t) we only keep the zeroth order term in the expansion
of exp(±iky), 
then
we are looking at terms of similar magnitude. We write
called
the magnetic dipole Hamiltonian, and
called
the electric quadrupole Hamiltonian.
Transition induced by WDM obey the magnetic dipole transition selection rules
or, if H0 includes a spin orbit coupling term,
Transition induced by WQM obey the electric quadrupole transition selection rules
Auf diesem Webangebot gilt die Datenschutzerklärung der TU Braunschweig mit Ausnahme der Abschnitte VI, VII und VIII.
