Charakterentabelle für Punktgruppe Th
=exp(2
i/3)=exp(2i/3)| Th | E | 4C3 | (4C3)2 | 3C2 | i | (4S6)5 | (4S6) |  h |
Rotation |
Fkt. |
|
|---|---|---|---|---|---|---|---|---|---|---|---|
| Ag | +1 | +1 | +1 | +1 | +1 | +1 | +1 | +1 | - | x2+y2+z2 | - |
| Eg | +1 +1 |
+ + * |
+* + |
+1 +1 |
+1 +1 |
+ + * |
+* + |
+1 +1 |
- | (x2-y2, 2z2-x2-y2) | - |
| Tg | +3 | 0 | 0 | -1 | +3 | 0 | 0 | -1 | (Rx, Ry, Rz) | (xy, xz, yz) | - |
| Au | +1 | +1 | +1 | +1 | -1 | -1 | -1 | -1 | - | - | xyz |
| Eu | +1 +1 |
+ + * |
+* + |
+1 +1 |
-1 -1 |
- - * |
-* - |
-1 -1 |
- | - | - |
| Tu | +3 | 0 | 0 | -1 | -3 | 0 | 0 | +1 | (x, y, z) | - | (x3, y3, z3) (xy2, x2z, yz2) (xz2, x2y, y2z) |
Th
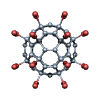
C60Br24
| Anzahl der Symmetrieelemente | h = 24 |
| Anzahl der irreduziblen Darstellungen | n = 8 |
| Anzahl der reellen irreduziblen Darstellungen | n = 6 |
| abelsche Gruppe ? | nein |
| chiral ? | nein |
Auf diesem Webangebot gilt die Datenschutzerklärung der TU Braunschweig mit Ausnahme der Abschnitte VI, VII und VIII.