This chapter begins with a mathematical article. People who want to
know more about benzene should click here.
If we measure n-energy state (for instance, of a particle in a potential box)
we will have the following mathematical expression
| H |n> = | En | |n> | Hyn = | En yn | |
| Energy operator ¾ . | ¾¾¾¾ Eigenfunction | ¾¾ | |||
| ¾¾¾¾ Eigenvalue ¾¾¾¾ | |||||
Applying <m| (Eigenfunction) and correspondingly integrating ò ym*.....dV the initial
formula one will have
| <m|H|n> = En <m|n> | ò ym* Hyn dV = En ò ym* yn dV | |
| = En δm,n . | = En δm,n . |
We can write down quantities <m|H|n>
and ò ym* yn dV o=in a shorter matrix form Hmn
that includes only diagonal components in the case of eigenfunction. And other
components are equal to 0:
| <m|H|n> = Hmn ≡ | æ ç ç ç ç ç ç è |
H11 | H12=0 | ... | H1n=0 | ü ú ú ú ú ú ú ø |
= | æ ç ç ç ç ç ç è |
E1 | 0 | ... | 0 | ü ú ú ú ú ú ú ø |
| H21=0 | H22 | ... | H2n=0 | 0 | E2 | ... | 0 | ||||||
| ... ... ... |
... ... ... |
... ... ... |
... ... ... |
... ... ... |
... ... ... |
... ... ... |
... ... ... | ||||||
| Hn1=0 | Hn2=0 | ... | Hnn | 0 | 0 | ... | En |
In this case, the diagonal matrix components give energy eigenvalues of the system under consideration. However, the background is that |n> and correspondingly |m> are eigenfunctions. What does this system of equations look like when we know only improper functions? Let's analyse our system in the following way: let's expand our eigenfunction |n> into other basis states, moreover the corresponding basis vectors |i> will have their own eigenfunction H|i>.
|n> = Σi ai|i> yn = Σi ai φi
Substituting it into our Schroedinger equation (with En = E):
H|n> = E|n> H yn = E yn
H Σi ai|i> = E Σi ai|i> H Σi ai φi = E Σi ai φi
Application of <k| and correspondingly òfk*...dV (<k| is orthogonal to |i>) will give us
Σi ai <k|H|i> = E Σi ai <k|i> Σi ai òfk* Hφi dV = E Σi aiòfk* φi dV
Σi ai Hki = E ak Σi ai Hki = E ak
This is the linear set of equations:
| i → | a1 H11 | + | a2 H12 | + | a3 H13 | + | ... | = E a1 |
| ¯ k | a1 H21 | + | a2 H22 | + | a3 H23 | + | ... | = E a2 |
| a1 H31 | + | a2 H32 | + | a3 H33 | + | ... | = E a3 | |
| . . . |
. . . |
. . . |
. . . |
This a well-known set of equations:
| . | = E . | ||
| Matrix¾ | ¾Vector¾ | ||
There are particular algebraic ways to determine eigenvalues E and use transformation which gives us eigenfunction (here eigenvectors).
We will describe a simple system with only 2 states
which, however, can give us basic knowledge of spectroscopy and chemistry. For
instance, let's have a look at benzene that has the following electronic
structure:
| Benzene ≡ |  |
|Benzene> = a1|1> + a2|2>
H |Benzene> = E |Benzene> |
Multiplication by <1|:
a1 <1|H|1> + a2
<1|H|2> = a1 E
and correspondingly <2|:
a1 <2|H|1> + a2
<2|H|2> = a2
E
Applying corresponding abbreviation <1|H|1> = H11 and so on, we will have the
following result:
| H11 a1 + H12 a2 = E a1 | ® | a1/a2 = H12/E − H11 | }equal |
| H21 a1 + H22 a2 = E a2 | ® | a1/a2 = E − H22/H21 |
® H12 · H21 = (E − H22) ( E − H11)
E2 − (H22 + H11) E = H12 · H21 − H11 · H22
→
| EI,II = H11 + H22/2 ± [(H11− H22)²/4 + H12 H21]½ |
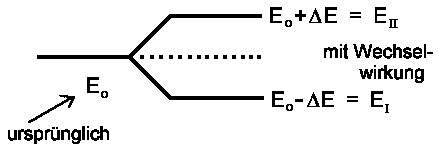
There are two stationary energy states EI and EII. If H12 = H21 = 0 there are no interactions between states |1> and |2> and then EI = H11 ≡ E1 and EII = H22 ≡ E2. That' why EI and EII correspond to energyvalues of unperturbed states:
H12 = H21 = 0 → EI,II = (H11 + H22)/2± (H11− H22)/2
The formulas will be simpler if terms H11 and H22 are
equal (as for benzene): H11 = H22 = E0. And if
we assign interaction H12 = H21 by ΔE then the
energy of stationary states |I> and |II>:
| EII,I = E0 ±DE |
 |
We have energy of stationary states |I> and |II>. And how does the
wavefunction look? Now we can construct the wavefunction from known
wavefunctions of states |1> and |2> in the example considered
(H11 = H22 = E0 and H12 =
H21 = −ΔE). The solution is as follows:
| |I> = 1/Ö2(|1> + |2>) |II> = 1/Ö2(|1>- |2>) |
Now let's have a look at normalization, orthogonalization and diagonal energy matrix:
a) Normalization:
= ½ (<1|1> + <2|1> + <1|2> + <2|2>) = ½ (<1|1>-<2|1>-<1|2> + <2|2>)
= ½ (1 + 0 + 0 + 1) = ½ (1 − 0 − 0 + 1)
<I|I> = 1 <II|II> = 1
b) Orthogonalization:
<I|II> = 1/Ö2(<1| + <2)1/Ö2(|1> - |2>)
= ½ (<1|1> + <2|1> -<1|2>-<2|2>)
= ½ (1 + 0 − 0 − 0 − 1)
® <I|II> = <II|I> = 0 (als Übung beweisen)
c) The energy matrix ![]() must be
for eigenvectors |I>, |II> diagonal components:
must be
for eigenvectors |I>, |II> diagonal components:
HI,I = <I|H|I> = ½ (<1| + <2|) H (|1> + |2>)
= ½ (H11 + H21 + H12 + H22) = ½ (E0 −ΔE −ΔE + E0) = E0−ΔE = EI
HI,II = <I|H|II> = ½ (<1| + <2|) H(|1>- |2>)
= ½ (H11 + H21 − H12 − H22) = ½ (E0 − ΔE + ΔE − E0) = 0
HII,I = <II|H|I> = ½ (<1| -<2|)H(|1> + |2>)
= ½ (H11 − H21 + H12 − H22) = ½ (E0 + ΔE −ΔE − E0) = 0
HII,II = <II|H|II> = ½ (<1| -<2|)H(|1>- |2>)
= ½ (H11 − H21− H12 + H22) = ½ (E0 + ΔE + ΔE + E0) = E0 + ΔE = EII
In general case H11 ¹ H22, H12 ¹ H21 are stationary states |I>, |II> having initial states |1> and |2>
|I> = |1> a1I + |2> a2I (for EI) |II> = |1> a1II + |2> a2II (for EII)
Complex constants have the following relationships:
a1I/a2I = H12/(EI - H11) |a1I|2 + |a2I|2 = 1
a1II/a2II = H12/(EII - H11) |a1II|2 + |a2II|2 = 1
Here one can find few simple chemical applications of above-mentioned for ammonia, H2+ and H2.
Bindings of different ions with electron
Two atomic asymmetric molecules often have weak bindings. Why?
If we consider, for instance, proton and positive Li ion. So now initially terms H11 and H22 are different and |H11− H22| >>DE. Then it will be (for H12 = H21 = −ΔE):
EI,II = (H11+H22)/2 ± (H11−H22)/2[1 + 4ΔE²/(H11−H22)²]½
Since x = 4ΔE2/(H11−
H22)2 is smaller than 1 and in the first approximation is
(1 + x)½ ≈ 1 + x/2
one will have:
| EII = H22 + ΔE²/(H22−H11) |  |
| EI = H11 -DE²/(H22−H11) |
The additional energy fragmentation "exchange energy" ΔE²/(H22−H11) is smaller than that of H2+ in this case.
(EII−EI)/(EII−EI)H2+ = 2ΔE²/(H22−H11)/2ΔE = ΔE/(H22−H11) << 1
![]()
Auf diesem Webangebot gilt die Datenschutzerklärung der TU Braunschweig mit Ausnahme der Abschnitte VI, VII und VIII.